HEDRAWEB

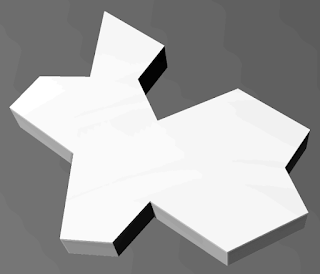
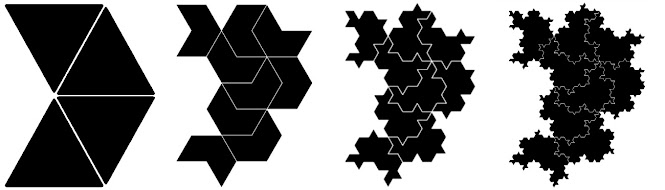
All permutations of polykites from 1-24 have been exhaustively researched by Joseph Myers.  His computational results almost run into trillions.  For more info, please visit - https://www.polyomino.org.uk/mathematics/polyform-tiling/ I have already covered a few polykites (including the hat and turtle) and have now picked out another that has an interesting property.  This polygon has sixteen sides and I have named it the 'hare'.  It also resembles a bushy tailed squirrel but I already have accounted for two of them - https://hedraweb.wordpress.com/2022/10/14/polydrafter-squirrels/ It tiles periodically with or without reflections Hares can be assembled to make up triangles of unlimited size.  The hexagon on the right is made up of six of the smallest triangles. Smallest hexagon inside a large triangle.   The triangular area above can also be assembled in at least one other way.  I flipped it to suit.   Below is a larger hexagon made up ...

I started with a chevron consisting of four equilateral triangles.  Each of those was then subdivided into nine smaller triangles, so that alternating bumps and notches could be applied to the centre of each side on the perimeter (for practical reasons I used a reverse approach, scaling up sides by a factor of three and reducing once done).  In theory, this process can continue indefinitely to produce complex fractal-like outlines.     There are left and right versions of the modified chevron, depending on which face is upward.  This means you cannot use both versions in the same tessellation.  Nine copies of each newly generated tile, can be put together to produce the next iteration.  Applying bumps and notches to even numbered polyiamonds, alters the tiling properties after the first iteration. There are four permutations for the chevron but only two for the modified versions, i.e., first and third from the left. These bumped and notched chevro...