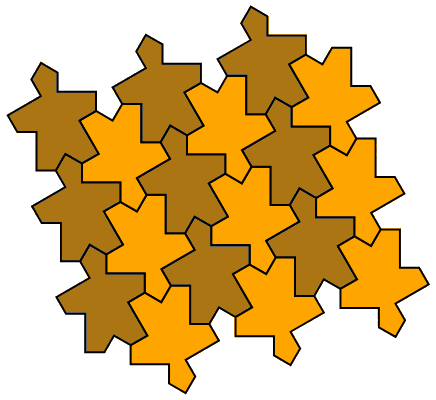
The gingerbread man

-

Ortwin Schenker named this shape the gingerbread man.  It can only tile one way but hides a secret or two.

This is one from hundreds of computer generated polygons supplied by Bob Nungester and Bram Cohen.  The shapes are all based on the Spectre, i.e., side lengths of one or two units and with similar angles. 



I pulled out a couple of other tiles (below, far right) that coincidentally make up the top and lower half of the gingerbread man.  Both display mirror symmetry and can tessellate in various ways.  I later named them 'yin' and 'yang'





These two polygons tile in similar ways.  There are six combinations of central cores (rotations of two or more tiles pivoting around a central point) for each.  First we have yin:



And next we have yang:




Interesting periodic patterns can be created easily by combining zigzag motifs.




Also, more chaotic patterns can evolve from periodic strips.






Below is a patch of yin with a yang void.







And another patch but this time of yang with a yin void.






Curiously yin and yang have exactly the same areas.  How do I know this?  Well, by splitting the gingerbread pieces further from top to bottom and then mirroring one half of them, they produce an identical shape.






But this polygon is less interesting unless flipping of the tile is allowed.



Extra possibilities with mirroring,





Yin and yang can also produce tilings from a void of this new shape.  As with all of these examples, patches should be able to extend indefinitely.






Below are a couple of radial patterns displaying 3-fold and 2-fold rotational symmetry.











Here are two radials that display the same central cores but with different 'outer' rings.









The example below has been rotated through 90 degrees for artistic reasons.  It now reminds me of the beautiful gas giant, Jupiter.







Most of these drawings were made up in a specially adapted tiling applet by French mathematician Arnaud Chéritat (very much appreciated).  Below is a link to it and two others for the hat/turtle and Spectre.

https://www.math.univ-toulouse.fr/~cheritat/AppletsDivers/Monotile/generic/

https://www.math.univ-toulouse.fr/~cheritat/AppletsDivers/Monotile/v2/

https://www.math.univ-toulouse.fr/~cheritat/AppletsDivers/ChiralMonotile/

Comments

Post a Comment

Popular posts from this blog

Determining chess 960 starting positions with a standard die (no rethrows)

-
Image
Chess 960 is a chess game variant invented by Bobby Fischer in 1996.   In Chess 960, the pawns are lined up on the second rank as in standard chess.   The first rank pieces are positioned randomly whilst conforming to certain caveats - the king must be placed somewhere between the rooks to allow castling; the bishops must stand on opposite coloured squares. I've worked out a system that allows me to select a starting arrangement for the first rank pieces using a standard six sided die without the need for rethrows.  My dice procedure is different from that of  I ngo Althöfer's (devised in 1998), where rethrows can occur.   Some information on how his system works can be seen here https://www.chessvariants.org/diffsetup.dir/fischer-random-setup.html The die I use to generate the random piece arrangement is a standard cube/hexahedron (one of the platonic solids).  O nly five or six throws of the die are needed to formulate one of the 960 ...
Read more

The golem

-
Image
This shape which I have named the golem, was hand picked from hundreds of Spectre-like polygons (Spectroids) that were sent my way via Ortwin Schenker, Bob Nungester and Bram Cohen.   This golem has the same number of sides as the Spectre and also shares internal angles of 90, 120 and 270 degrees. Below left is the simplest of the periodic tilings.  Positioning of new tiles is forced in most cases.  That said, the pattern can deviate at any time by placing other rotations where there are choices (as shown in the example bottom right). The golem is a delight to tessellate (you can't go wrong!).  Below are a few more examples of periodic tilings.  These can be sewn together to make larger chaotic tilings with a bit of tweaking. Next up are a couple of radial patterns.  The example on the right is (I think) quite unusual in that three golem segments are pointing towards the centre whilst the other three are pointing outwards. The golem has mirror symmetry...
Read more

Fractal chevron

-
Image
I started with a chevron consisting of four equilateral triangles.  Each of those was then subdivided into nine smaller triangles, so that alternating bumps and notches could be applied to the centre of each side on the perimeter (for practical reasons I used a reverse approach, scaling up sides by a factor of three and reducing once done).  In theory, this process can continue indefinitely to produce complex fractal-like outlines.     There are left and right versions of the modified chevron, depending on which face is upward.  This means you cannot use both versions in the same tessellation.  Nine copies of each newly generated tile, can be put together to produce the next iteration.  Applying bumps and notches to even numbered polyiamonds, alters the tiling properties after the first iteration. There are four permutations for the chevron but only two for the modified versions, i.e., first and third from the left. These bumped and notched chevro...
Read more